Fractal Home
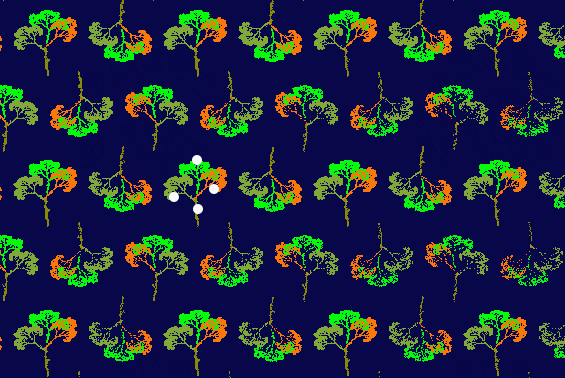
Fractal tesselation of the Euclidean plane of type PGG
Producing Wallpapers
You can produce your own endless background images with the Wallpaper Machine: Choose an IFS figure and the wallpaper mode. Form the IFS by moving a single fixed point or move the whole IFS (Group Move Points) to another position of the wallpaper machine. Both influences the result. Do a screenshot and cut the tesselation for your background image like the example shown above.
Some hints:
With an IFS, which produces a figure of high density, the tesselation of the Euclidean plane looks nicer, if the tesselation
elements don't overlap each other. By moving the fixed points to shorter distances You can make the IFS smaller. It is also
possible to adjust the tesselation zoom factor in order to give more room to the base figure. IF the basic figure is thin,
overlapping could be a good idea to produce exciting tesselations.
Names of the Wallpaper Groups
A wallpaper ornament fills the whole Euclidean plane with a basic pattern. 17 different modes are possible and therefor used, described by cryptic names like CMM, P31M and P6M. Watch the results to understand how the transformations work. The following table explains the symbols used to describe these transformations.
| Symbol | Meaning | |
|---|---|---|
P | The letter P means that there is a primitve cell. |
|
C | The letter C is used to say that there is a rhombic cell with at least one diagonal as a mirror axis. The cell could be integrated into a double sized rectangle cell, positioned as a centered cell. |
|
M | The letter M tells us that there is a mirror transformation. |
|
G | The letter G announces a glide reflection. A glide reflection is a mirror reflection followed by a vector move. |
|
2, 3, 4, 6 | The numbers tell us about centers of a n-ordered rotation symmetry. Only these numbers are possible. An example: The number 3 says, that a rotation of 120° will leave the pattern unchanged (3×120° = 360°). |
|
1 | The number 1 in P31M (between 3 and M) signalizes that not all rotation centers with an angle of 60° are located on an reflection axis. In P3M1 all such rotation centers are located on an reflection axis. |
|
